Slide Rules
A primary computing tool for engineers, used until
electronic pocket calculators became popular in 1980s.
Simple logarithmic one-scale grid was invented around 1620 by E. Gunter,
it's used even today for mapping and navigation purposes. In 1632 William
Oughtred added secondary scale to make a simple rule. Additional
scale enhancements were made by E. Wingate and S. Patridge. In today's
form slide rule was invented by A. Manheim in 1850. In XX century
Darmstadt introduced Log-Log scales, later Ritz added 3 trigonometric
scales and inverted scale.
A typical linear slide rule consists of a set of scales, these scales are placed in moving part (a middle one) and outer part. Usually there's also a transparent moving part called cursor, with a vertical line which allows to point a number in a slider.
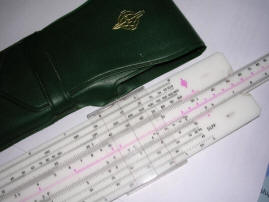
Logarex 27402-III
Typical slide rule from 1970s, made in Czechoslovakia. It has all
typical scales. Trigonometric scales are on the reverse of slider. In
some scales you can see "Cu", "Al" and similar markings. These are for
computing amount of power which can be drawn from a cable made of
specific alloys. It was a good aid for power engineering appliances.
It's made of white plastic, quite durable but it can get stuck if not
handled properly, then it needs to be moved back and forth few times.
|
|
|
|
| General view with cover | Cursor | Markings |
| Hemmi Bambou - Patent 58115 This Japanese slide rule was used by my Grandfather. It has all basic scales plus trigonometric on reverse of slider. It's made of bamboo wood, so it is self-lubricating and it still slides smoothly without jams. Cursor is made of metal and celluloid. It was manufactured between 1917 and 1927. But some other sources state that these cursors were used until 1930s. Hemmi, from Jiro Hemmi, was a well known brand in manufacturing these instruments. In 1910 they started manufacturing slide rules from bamboo wood, making it self-lubricating and preserving geometry. In 1912 Hemmi got a Japanese patent for a slide rule made of wood with laminated, celluloid scale. In 1917 they started to use Sun trademark for foreign market. In 1928 company changed its name to Hemmi Seisakusho & Co. Then they started labelling cursors "Sun Hemmi". In 1930s they manufactured thousands rules every year. Since 1928 some Hemmi rules have P and Q scales. Seen on a few photos, but I have no idea what they could be used for. As most Japanese companies, Hemmi's business disappeared with starting of world war II. Some small quantities were manufactured until 1950s (last ones even in 1970s), but it was not a big production. More: MANUAL, incomplete, and in strange format (photo of a museum item). |
|
|
|
|
|
| With cover | Cover detail | Cursor |
|
|
|
|
| Some conversion tables. Notice Polish "Gerlach", it's not cutlery manufacturer but Gustaw Gerlach - clockmaker. | Under the slider | Trigonometric scales |
If you want to know Hemmi's history, I recommend
visiting
this page.
If you want more history, you can take a look at
archived copy of Japanese slide rules page. Nice timeline of Hemmi's
business.
Pocket slide rule
This small slide rule was used even in high schools as simple
calculator. It's probably from 1960s of 70s.
|
|
|
| With cover | 2*9=... |
[added: 2016-03-12]
"SP" Soviet slide rule
Soviet slide rule made in 1953. It is made of wood coated with
celluloid, so it has good lubrication and doesn't get stuck. The pointer
is made of metal with celluloid window. It was made in Leningrad (today
Saint Petersburg), by "Factory of computing instruments", being a
"Industrial division of Lengorispolkom", according to markings.
Lengorispolkom is the executive comitee of Leningrad city. The rule has
normal, trigonometrical and S-T scales (on the other side of slider). It
has also a normal ruler for quick drawing and table of typical
engineering constants on the reverse.
The logo present on the box and rule is not known, probably it has some
relations to "CCCP".
[added: 2016-03-12]
Skala "SLPP" Slide Rule
A Polish slide rule made in 1960s. It is made of plastic, so lubrication
is not perfect and currently it doesn't move as slightly as wooden ones.
It is quite long and has many scales in front, but no scales on the rear
or slider's reverse. All scales are typical x, x^2 and 1/x scales, x^3,
Square root, logarithmic, trigonometric (sin, tg, S-T) and special "M"
scale for computing of lengths in right-angled triangle.
Manual [in Polish]: Page 1, Page 2.
Typical scales are:
- A and B (labelled X) logarithmic scales (1 - 10, sometimes labelled as "right
1"), placed one on slider one on outer part, used to divide and multiply
numbers.
- C and D logarithmic scales, 2-decade (labelled X2), one on
slider, one on outer part.
- G scale (1/X) located on slider - used to compute inversions
- F (or L, Log) - linear scale for finding logarithms
- E (or Ln) - ex scale for finding exponentials
- LL scales - used to find logarithms and exponentials
- S, T and ST trigonometric scales, located on sliding part (popular in
eastern block rules) or outer.
If you compute using slide rule remember to get along with decimal places of the result!
Simple computations:
2. Division: a/b=x
a. Mark a on scale A with cursor.
b. Move scale B to set b under cursor
c. Read result from scale A under "1" (or "right 1" - if division by <1
used) of scale B.
3. Inversions - Inversion of number on scale B is a number on scale G. Simple. Or just divide 1/x.
4. Square: a^2=x
a. Mark a on scale A with cursor
b. Read square from scale D (under cursor)
5. Any power: ab=x
a. Use ex scale, set 1 of "X" scale in a in ex
scale.
b. Find b on "X" scale, read result on ex scale under it.